
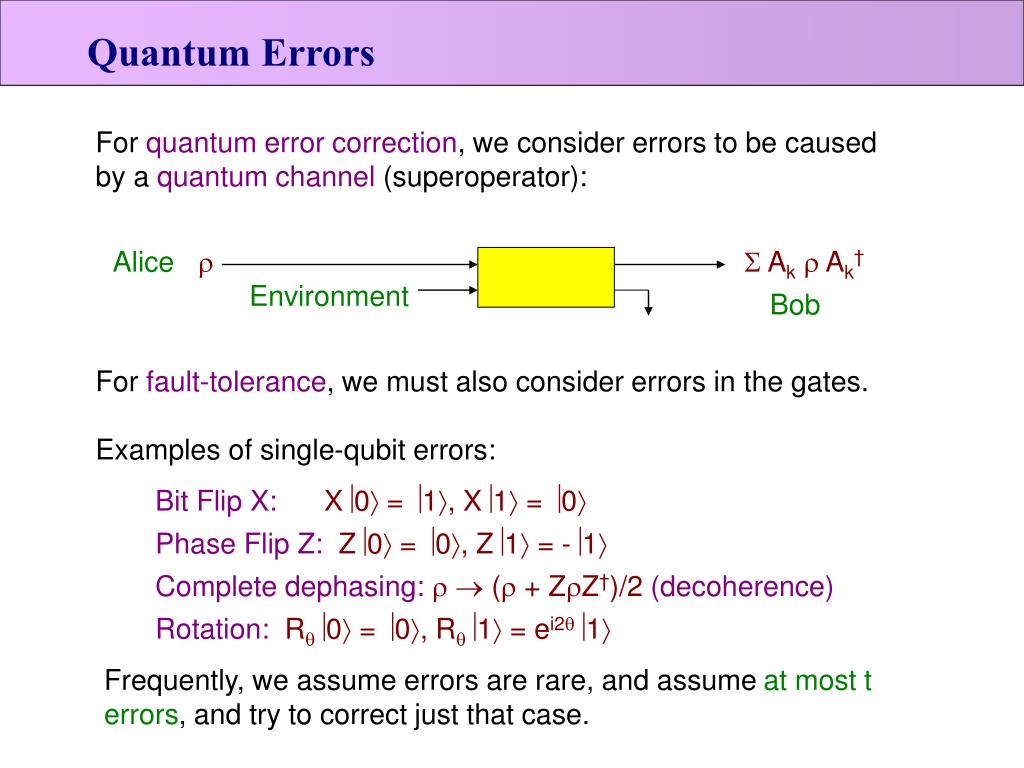

In classical information theory we would describe such a way of storing information as an 'encoding.' Thus we describe this strategy as using 'quantum codes'. Second, we want the information to be stored in a non-local manner. 1.2.1 The double-slit experiment 1.3 Superpositions 1.4 Interferometers 1.5 Qubits, gates, and circuits 1.6 Quantum decoherence 1.7 Computation: deterministic, probabilistic, and quantum 1.8 Computational complexity 1.9 Outlook 1.10. First, we somehow want to add redundency. 1.1 Two basic rules 1.2 Quantum interference: the failure of probability theory. In practice one it is likely to introduce more gates than these - but if you are doing a "proof-of-principle" - then you only need to implement these three gates. There are two principles that we will apply. The Stinespring Theorem (Theorem 2.8) immediately shows that this implies T. In this article we survey the current landscape of quantum error correcting (QEC) codes, focusing on recent theoretical advances in the domain of noise-adapted QEC, and highlighting some key open questions. In this work we propose and demonstrate a general quantum algorithm to evolve open quantum dynamics on quantum computing devices. A stray magnetic field in the $\hat z$ direction would cause this state to evolve under the Hamiltonian The theory of quantum error correction provides a scheme by which the effects of such noise on quantum states can be mitigated, paving the way for realising robust, scalable quantum computers. The states $|\uparrow\rangle$ and $|\downarrow\rangle$ could, for example, be spin states of a defect in silicon. Where $\alpha$ and $\beta$ are arbitrary numbers. (9.45)).Based on linear algebra we can make the following two observations. We establish operator structure identities for quantum channels and their error-correcting and private codes, emphasizing the complementarity relationship.
#Steinspring quantum error correction generator#
|\psi\rangle= \alpha|\uparrow\rangle+ \beta |\downarrow\rangle, where the ith row corresponds to finite geometry representation of the generator g i (see Eq. These are vulnerable to environmental noise.įor example, consider a state of the form There is a fundamental problem with quantum computers: any possible speed-up requires quantum entanglement and superpositions.


 0 kommentar(er)
0 kommentar(er)
